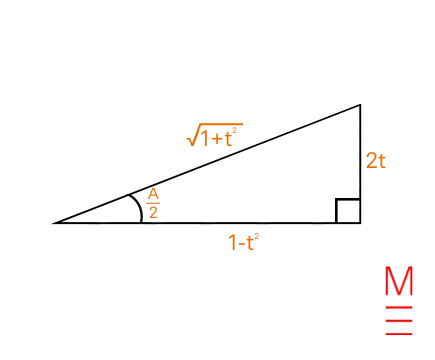The NESA Maths Reference Sheet is a superb useful resource… if you know the way to make use of it! Navigate features (together with trigonometric ones!) and relations with our Final NESA Maths Reference Sheet Information.
Whereas memorisation has its place in studying, Matrix recommends that college students be taught to derive their responses and learn to apply these formulae appropriately. As a bonus, we’ve included a nifty HSC Maths Cheatsheet so that you can obtain and print out!
Click on on the next formulation to see what they imply and apply them to a apply query!
| Capabilities |
| (x=frac{-b± sqrt{b^2-4ac}}{2a})
(textual content{For} ax^3 + bx^2 +cx + d = 0 😉 |
| Relations |
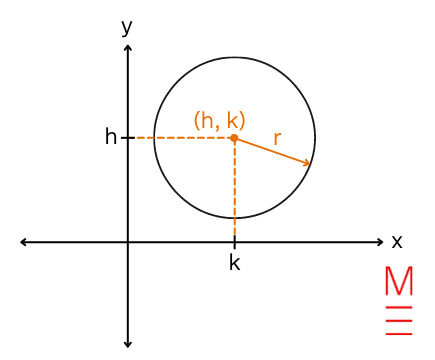
| ((x-h)^2+(y-k)^2=r^2) |
| Logarithmic and Exponential Capabilities |
| (textual content{log}_a a^x = x = a^{textual content{log}_a x})
(textual content{log}_a x = frac{textual content{log}_b x}{textual content{log}_b a}) (a^x = e^{xtext{In}a}) |
| Trigonometric Capabilities |
| ( textual content{sin}A = frac{textual content{opp}}{textual content{hyp}}, textual content{cos}A = frac{textual content{adj}}{textual content{hyp}}, textual content{tan}A = frac{textual content{opp}}{textual content{adj}})
(A= frac{1}{2}abtext{ sin}C) (frac{a}{textual content{sin}A} = frac{b}{textual content{sin}B} = frac{c}{textual content{sin}C}) (c^2=a^2+b^2-2abtext{ cos}C) (textual content{cos}C = frac{a^2+b^2-c^2}{2ab}) (l=r theta) (A=frac{1}{2}r^2theta)
Trigonometric identities (textual content{sec}A = frac{1}{textual content{cos}A}, textual content{cos}A ≠0) (textual content{cosec}A = frac{1}{textual content{sin}A}, textual content{sin}A ≠0) (textual content{cot}A = frac{textual content{cos}A}{textual content{sin}A}, textual content{sin}A ≠0) (textual content{cos}^2x+textual content{sin}^2x=1)
Compound angles (textual content{sin}(A+B)=textual content{sin}Atext{cos}B+textual content{cos}Atext{sin}B) (textual content{cos}(A+B)=textual content{cos}Atext{cos}B-text{sin}Atext{sin}B) (textual content{tan}(A+B)=frac{textual content{tan}A+textual content{tan}B}{1-text{tan}Atext{tan}B}) (textual content{If } t=textual content{tan} frac{A}{2} textual content{ then } ) (textual content{cos}Atext{cos}B=frac{1}{2}[text{cos}(A-B)+text{cos}(A+B)]) (textual content{sin}Atext{sin}B=frac{1}{2} [text{cos}(A-B) -text{cos}(A+B)]) (textual content{sin}Atext{cos}B = frac{1}{2} [text{sin}(A+B)+text{sin}(A-B)]) (textual content{cos}Atext{sin}B=frac{1}{2}[text{sin}(A+B)-text{sin}(A-B)]) (textual content{sin}^2nx=frac{1}{2}(1-text{cos}2nx)) (textual content{cos}^2nx=frac{1}{2}(1+textual content{cos}2nx)) |
Capabilities
| Use | Formulation | Variables |
| Clear up quadratic equations | (x=frac{-b± sqrt{b^2-4ac}}{2a}) | a, b and c are the coefficients of a quadratic equation within the type:(ax^2+bx+c=0) |
Again to prime
Subsequent part: Relations
Instance 12:
Contemplate the next equation: (-2y^2+3y+1=0)
Discover all of the options of (y).
Resolution 12:
| Although the above equation makes use of (y) as a variable as an alternative of (x), the quadratic system works the identical approach.
start{align*} Therefore, substitute (a=-2), (b=3) and (c=1) into (y=frac{-b± sqrt{b^2-4ac}}{2a}): start{align*} |
Again to prime
| Use | Formulation | Variables |
| Cubic roots | start{align*} textual content{For} ax^3 + bx^2 +cx + d &= 0: α+β+γ &= – frac{b}{a} αβ+αγ+βγ &= frac{c}{a} textual content{and } αβγ &= -frac{d}{a} finish{align*} |
α, β and γ are roots of a cubic equation within the type: (ax^3 + bx^2 +cx + d = 0) |
Again to prime
Instance 13:
(x=1) and (x=3) are two options to the monic equation: (ax^3 + bx^2 +cx + d = 0)
On condition that the product of the roots is -6, discover the coefficients (a,b,c) of this cubic polynomial.
Resolution 13:
| The query states that the equation is monic. Which means, a=1.
start{align*} αβγ &= -frac{d}{a} α+β+γ &= – frac{b}{a} αβ+αγ+βγ &= frac{c}{a} It’s on condition that (x=1) and (x=3) are options to the cubic equation, (x=1) and (x=3) are two roots of the equation. Therefore, substitute (α=1) and (β=3) into (αβγ = -6): start{align*} To search out the coefficients b and c (we’ve already discovered (a=1) and (d=6)): Substitute (α=1), (β=3) and (γ=-2) into equation 1: (α+β+γ = -b) and equation 2: (αβ+αγ+βγ = c): start{align*} textual content{Equation 2: } (1)(3)+(1)(-2)+(3)(2) &= c Due to this fact, the coefficients of the equation (ax^3 + bx^2 +cx + d = 0) are (a=1), (b=-2),(c=7) and (d=6). |
Again to prime
Relations
| Use | Formulation | Rationalization |
| Cartesian equation of a circle | ((x-h)^2+(y-k)^2=r^2) | Cartesian equation of a circle centred at (h, ok) with radius = r
|
Again to prime
Subsequent part: Logarithmic and Exponential Capabilities
Instance 14:
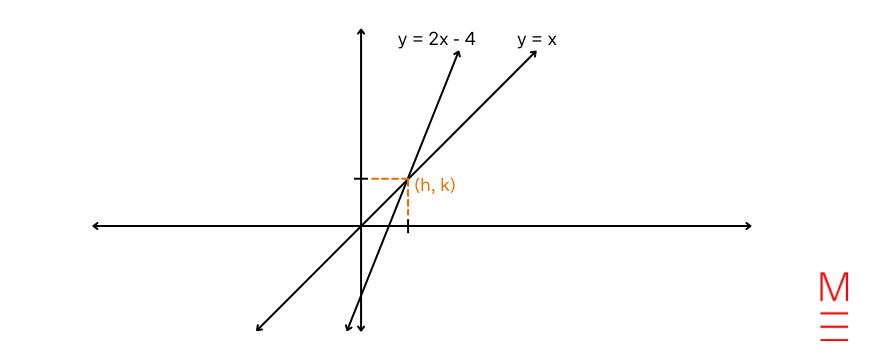
The centre of a circle lies on the intersection of two traces: (y = x) and (y = 2x – 4)
On condition that the realm of this circle is (16π ) items2, discover the Cartesian equation of this circle.
Resolution 14:
| To search out the centre of the circle, first we concurrently clear up the equations (y = x) and (y = 2x – 4) to seek out their level of intersection.
start{align*} textual content{Substitute } x &= 4 textual content{ into } y = 2x – 4 : ⇒ textual content{Centre of circle (h, ok) } & = (4, 4) To search out the radius r of the circle, we are able to use the data that the realm of this circle is (16π ) items2. The system for the realm of a circle is: ( A = pi r^2 ) start{align*} The equation of the circle is: ((x-h)^2+(y-k)^2=r^2) the place ((h, ok)) = ((4, 4)) and (r = 4) start{align*} |
Again to prime
Logarithmic and Exponential Capabilities
| Formulation | Software |
| (textual content{log}_a a^x = x = a^{textual content{log}_a x}) | Simplifying and manipulating expressions. |
| (textual content{log}_a x = frac{textual content{log}_b x}{textual content{log}_b a}) | Most calculators can calculate (textual content{log}_{10}) and (textual content{ln}). To enter logarithms with out base 10 or e, you need to use this system convert your logarithm into an expression involving logarithms with solely base 10 or e.
e.g. (textual content{log}_8 9 = frac{textual content{log}_{10} 9}{textual content{log}_{10} 8} = frac{ textual content{ln}9}{textual content{ln}8}) (textual content{log}_2 5 = frac{textual content{log}_{10} 5}{textual content{log}_{10} 2}= frac{ textual content{ln}5}{textual content{ln}2}) |
| (a^x = e^{xtext{ln}a}) | To combine expressions within the type (a^x):
start{align*} Evaluation your information of integration by substitution right here. |
Again to prime
Trigonometric Capabilities
| Use | Formulation | Rationalization |
| Trigonometric features | start{align*} textual content{sin}A = frac{textual content{opp}}{textual content{hyp}} textual content{cos}A = frac{textual content{adj}}{textual content{hyp}} textual content{tan}A = frac{textual content{opp}}{textual content{adj}} finish{align*} |
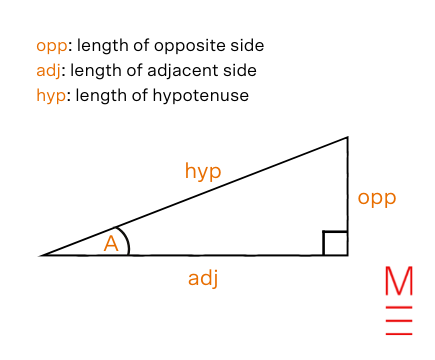 |
Again to prime
Instance 15:
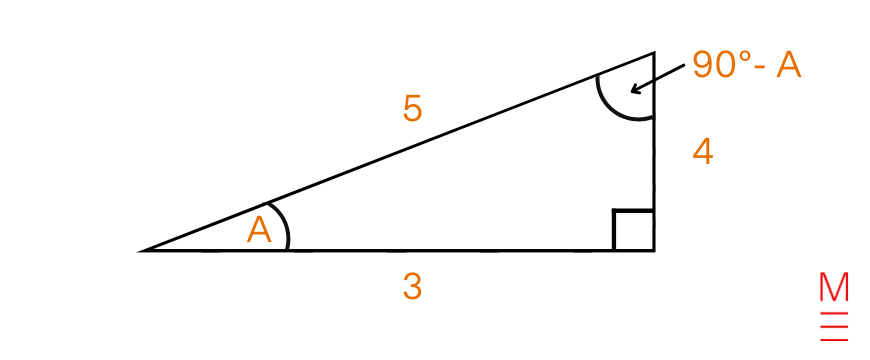
Given the beneath right-angle triangle, discover the worth of the next trigonometric expressions:
a) sinA
b) cos(90°-A)
c) tan90°
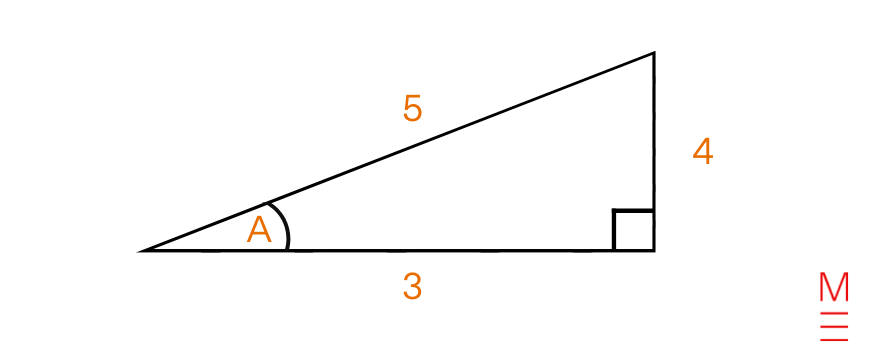
Resolution 15:
| a) sinA = (frac{4}{5})
b) cos(90°-A) = sinA = (frac{4}{5}) Alternatively, recognise that (90°-A) is an angle within the triangle.
Therefore, cos(90°-A) = (frac{textual content{adj}}{textual content{hyp}}) = (frac{4}{5}) c) tan90° = undefined As we are able to see on this proper angle triangle, the size reverse 90° is the hypotenuse. Therefore, the expression ( textual content{tan}90° = frac{textual content{opp}}{textual content{adj}}) is undefined. |
Again to prime
| Use | Formulation | Rationalization |
| Space of a triangle | (A= frac{1}{2}abtext{sin}C) | 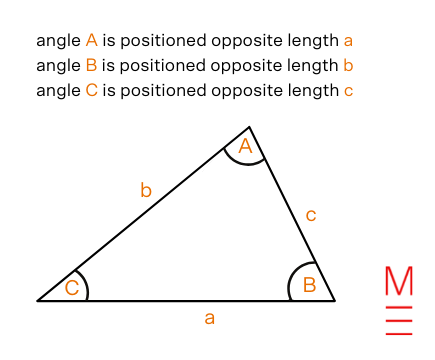 |
Again to prime
Instance 16:
On condition that the next triangle has an space of (4 sqrt{3}) cm2, discover the size x.
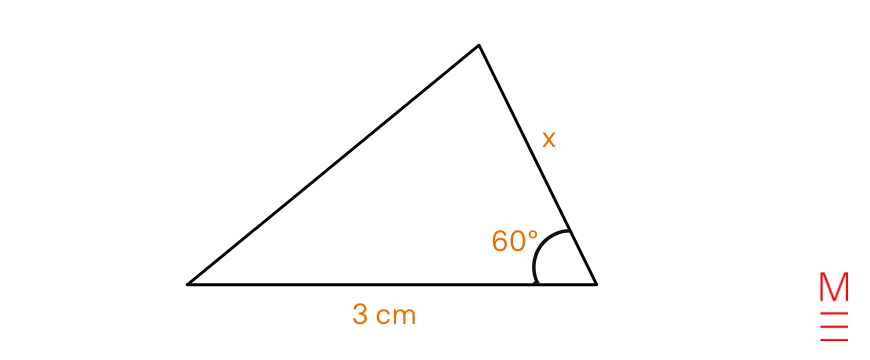
Resolution 16:
| Substitute (A= 4 sqrt{3}), (a=3), (b=x) and (C=60°) into (A= frac{1}{2}abtext{sin}C):
start{align*} |
Again to prime
| Use | Formulation | Rationalization |
| Sine rule | (frac{a}{textual content{sin}A} = frac{b}{textual content{sin}B} = frac{c}{textual content{sin}C}) |  |
| Cosine rule | (c^2=a^2+b^2-2abtext{cos}C)
This equation will also be rearranged as: (textual content{cos}C = frac{a^2+b^2-c^2}{2ab}) |
Again to prime
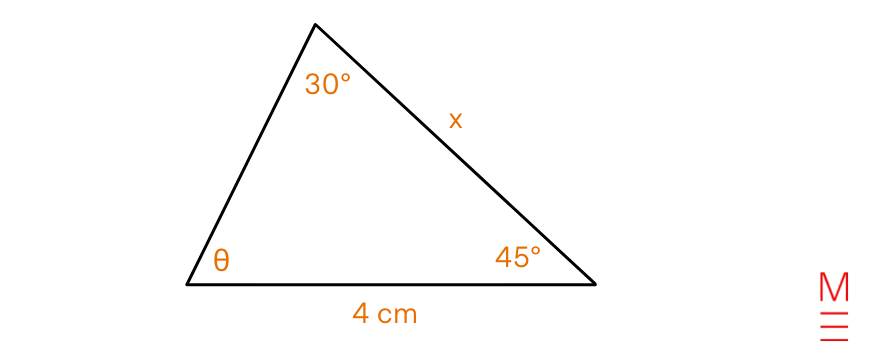
Instance 17:
Discover size x. Giver your reply to the closest 3 decimal locations.
The diagram is just not to-scale.
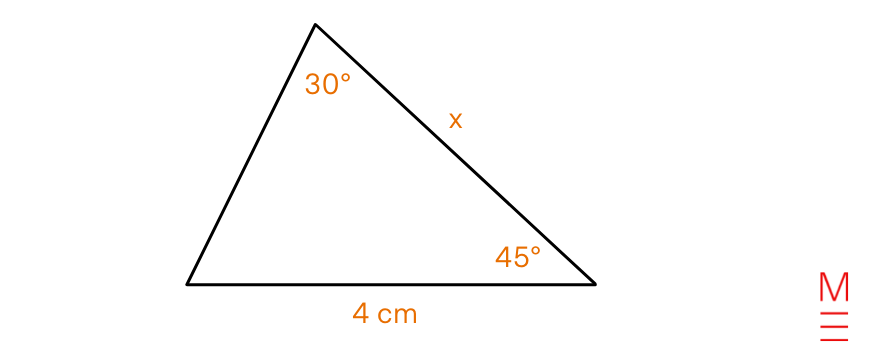
Resolution 17:
| With a purpose to use the sine rule to seek out x, we have to know the angle θ that’s reverse size x.
start{align*} Thus, we are able to use the sine rule: Substitute (a=4), (A=30°), (b=x), (B=θ=105°) into (frac{a}{textual content{sin}A} = frac{b}{textual content{sin}B}): start{align*} |
Again to prime
| Use | Formulation | Rationalization |
| Arc size of a sector
(utilizing θ in radians: ( pi ^c = 180°)) |
(l=r theta) | start{align*} l&= textual content{arc size} theta &= textual content{angle of sector in radians} r &= textual content{radius} finish{align*} 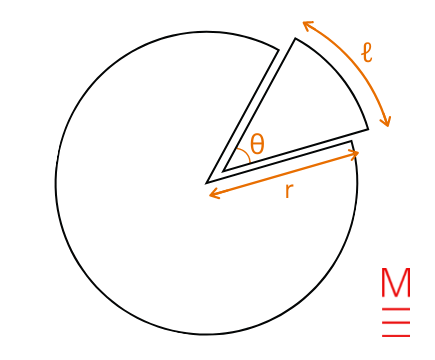 |
| Space of a sector
(utilizing θ in radians: ( pi ^c = 180°)) |
(A= frac{1}{2} r^2 theta) |
Again to prime
Instance 18:
a) Sal is fencing a paddock for his sheep. He desires to make the paddock the form of a sector with a radius of 6 metres. If he has (12 + 2π) metres of fencing, what’s the angle of the most important sector he can totally enclose? Give your reply as a precise worth in radians.
b) Therefore, discover the utmost space of Sal’s paddock.
Resolution 18 a):
The next diagram illustrates the paddock.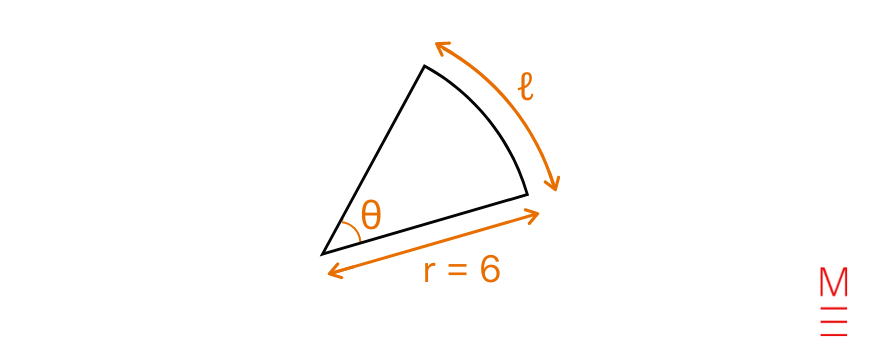 The perimeter of the sector: (P = r+r+l.textual content{ })Since Sal is restricted to ((12 + 2π)) metres of fencing, substitute (P =12 + 2π) and (r=6) (given).start{align*} The perimeter of the sector: (P = r+r+l.textual content{ })Since Sal is restricted to ((12 + 2π)) metres of fencing, substitute (P =12 + 2π) and (r=6) (given).start{align*}12 + 2π &= 6 + 6 + l l &= 2π finish{align*}Therefore, the arc size of the most important sector Sal can enclose is (2π). Nevertheless, this isn’t our last reply as we’re required to seek out the angle of the most important sector. Substitute (l = 2π) and (r=6) into (l=r theta):start{align*} 2π &= 6 occasions theta theta &= frac{π}{3} ∴ textual content{Angle of the most important sector} &= frac{π}{3}^c finish{align*} |
Resolution 18 b):
| We’re on condition that the radius of the sector is 6 metres and partly a), we discovered that the most important doable angle of the sector is (frac{π}{3}^c).
So, to seek out the most important space of the sector, substitute (r=6) and (theta=frac{π}{3}^c) into (A= frac{1}{2} r^2 theta): start{align*} |
Again to prime
Trigonometric identities
| Identification | Variables |
| (textual content{sec}A = frac{1}{textual content{cos}A}, textual content{cos}A ≠0) | Angle (A) can take any variable (aside from the place exceptions are particularly famous) and the identification will maintain true. |
| (textual content{cosec}A = frac{1}{textual content{sin}A}, textual content{sin}A ≠0) | |
| (textual content{cot}A = frac{textual content{cos}A}{textual content{sin}A}, textual content{sin}A ≠0) | |
| (textual content{cos}^2x+textual content{sin}^2x=1) |
Again to prime
Instance 19:
Show that: (textual content{tan}θtext{sin}θ + textual content{cos}θ = textual content{sec}θ)
Resolution 19:
| start{align*} LHS &= textual content{tan} theta textual content{sin} theta + textual content{cos} theta textual content{Since } textual content{tan} theta = frac{ textual content{sin} theta }{ textual content{cos} theta }, LHS &= frac{ textual content{sin} theta }{ textual content{cos} theta } occasions textual content{sin} theta + textual content{cos} theta &= frac{ textual content{sin}^2 theta }{ textual content{cos} theta } + frac{ textual content{cos}^2 theta }{ textual content{cos} theta } &= frac{ textual content{sin}^2 theta + textual content{cos}^2 theta }{ textual content{cos} theta } textual content{Since } textual content{sin}^2 theta + textual content{cos}^2 theta=1, &= frac{1}{ textual content{cos} theta } &= textual content{sec} theta &= RHS ∴ textual content{tan} theta textual content{sin} theta + textual content{cos} theta = textual content{sec} theta finish{align*} |
Again to prime
Compound angles
Increasing a sum of angles in a trigonometry operate
| Identification | Variables |
| (textual content{sin}(A+B)=textual content{sin}Atext{cos}B+textual content{cos}Atext{sin}B) | (A, B ∈ R)
Angle (A) and (B) can take any worth (they are often equal or unequal) and the identification will maintain true. |
| (textual content{cos}(A+B)=textual content{cos}Atext{cos}B-text{sin}Atext{sin}B) | |
| (textual content{tan}(A+B)=frac{textual content{tan}A+textual content{tan}B}{1-text{tan}Atext{tan}B}) |
Again to prime
Instance 20:
Discover the precise worth of (cos75º).
Resolution 20:
| start{align*} textual content{cos}75º &= textual content{cos}(45+30)º textual content{Since } textual content{cos}(A+B)=textual content{cos}Atext{cos}B-text{sin}Atext{sin}B textual content{cos}(45+30)º &= textual content{cos}45text{cos}30-text{sin}45text{sin}30 &= frac{ sqrt{2}}{2} occasions frac{ sqrt{3}}{2} – frac{ sqrt{2}}{2} occasions frac{1}{2} &= frac{ sqrt{6}}{4} – frac{ sqrt{2}}{4} ∴ textual content{cos}75º &= frac{ sqrt{6} – sqrt{2}}{2} finish{align*} |
Again to prime
T-formula
| Formulation | Rationalization |
| start{align*} textual content{If } t=textual content{tan} frac{A}{2} textual content{ then } textual content{sin}A &= frac{2t}{1+t^2} textual content{cos}A &= frac{1-t^2}{1+t^2} textual content{tan}A &= frac{2t}{1-t^2} finish{align*} |
(A ∈ R)
Substitute sinA, cosA or tanA with these ‘t-values’. That approach you gained’t should cope with trigonometric features, solely algebraic expressions involving t. Upon getting simplified your expression involving t, typically you will have to substitute (t=textual content{tan} frac{A}{2}) to seek out the values of (A).
Word: When A = 180°, (t=textual content{tan} frac{A}{2} = textual content{tan}90) is undefined. Meaning that you’ll want to individually verify whether or not A = 180° it’s a resolution if you use the t-formula. See Instance 21 beneath. |
Again to prime
Instance 21:
Given (2text{cos}2B – textual content{sin}2B = 1), clear up for (B), (0 le B le 180°):
If vital, give your solutions to the closest 2 decimal locations.
Resolution 21:
| start{align*} textual content{Let } t &= textual content{tan}B ⇒ textual content{sin}2B &= frac{2t}{1+t^2} textual content{cos}2B &= frac{1-t^2}{1+t^2} textual content{tan}2B &= frac{2t}{1-t^2} textual content{On condition that }2text{cos}2B – textual content{sinB}2B &= 1 |
Again to prime
Manipulating a product of trigonometric features right into a sum of trigonometric features
| Identification | Variables |
| (textual content{cos}Atext{cos}B=frac{1}{2}[text{cos}(A-B)+text{cos}(A+B)]) | Angle (A) and (B) can take any variable (they are often equal or unequal) and the identification will maintain true. |
| (textual content{sin}Atext{sin}B=frac{1}{2} [text{cos}(A-B) -text{cos}(A+B)]) | |
| (textual content{sin}Atext{cos}B = frac{1}{2} [text{sin}(A+B)+text{sin}(A-B)]) | |
| (textual content{cos}Atext{sin}B=frac{1}{2}[text{sin}(A+B)-text{sin}(A-B)]) |
Again to prime
Instance 22:
Discover the precise worth of the product of (textual content{cos}15º) and (textual content{sin}45º).
Resolution 22:
| start{align*} textual content{Since } textual content{cos}Atext{sin}B & =frac{1}{2}[text{sin}(A+B)-text{sin}(A-B)] ⇒ textual content{cos}15ºtext{sin}45º &= frac{1}{2}[text{sin}(15+45)-text{sin}(15-45)] &= frac{1}{2}[text{sin}(60)-text{sin}(-30)] &= frac{1}{2}[frac{ sqrt{3}}{2}+ frac{1}{2} ] ∴textual content{cos}15ºtext{sin}45º &= frac{sqrt{3}+1}{4} finish{align*} |
Again to prime
Double angle identities
| Identification | Variables |
| (textual content{sin}^2nx=frac{1}{2}(1-text{cos}2nx)) | (x, n ∈ R) |
| (textual content{cos}^2nx=frac{1}{2}(1+textual content{cos}2nx)) |
Again to prime
Instance 23:
Consider (2(textual content{sin}22.5º)^2). Give your reply in precise type.
Resolution 23:
| start{align*} textual content{Since } textual content{sin}^2nx &=frac{1}{2}(1-text{cos}2nx) ⇒ 2(textual content{sin}22.5º)^2 &= 2 occasions frac{1}{2}(1-text{cos}45º) &= 1- frac{ sqrt{2}}{2} &= frac{ 2- sqrt{2}}{2} ∴2(textual content{sin}22.5º)^2 &= frac{ 2- sqrt{2}}{2} finish{align*} |
Again to prime
,