Have you ever seen the 2021 HSC Arithmetic Superior (2 Unit) examination paper but?
On this submit, we are going to work our method via the 2021 HSC Maths Superior (2 Unit) paper and provide the options, written by our Head of Arithmetic Oak Ukrit and his staff. (Doing follow papers? See the options for the 2020 HSC Maths Adv Examination right here.)
Learn on to see tips on how to reply the entire 2021 questions.
Part 1. A number of Alternative
()
| Query | Reply | Resolution |
| 1. | B | start{align*} sin^2 5x + cos^2 5x &= 1 sin^2 5x &= 1 – cos^2 5x finish{align*} |
| 2. | C | start{align*} E(X) &= sum_{x = 1}^3 x P(X = x) &= (1)(0.6) + (2)(0.3) + (3)(0.1) &= 1.5 finish{align*} |
| 3. | D | Since a logarithm can solely settle for constructive enter, we have to be constrained by start{align*} 1 – x &> 0 – x &> -1 x &< 1 textual content{ or equivalently, } x in (-infty, 1) finish{align*} |
| 4. | C | Choices (A) and (D) present that there are near 100 downloads on day 1, which isn’t according to the graph given, so they’re fallacious. The graph given reveals that the variety of downloads will increase from day 1 to day 10, and it decreases after that. Possibility (B) reveals that the variety of downloads each day is roughly the identical, and that’s inconsistent with the graph given. The graph in possibility (C) is according to all of the options proven within the graph given. |
| 5. | A | Plugging in (x = 0) shortly offers the y-intercept as (y = 10(0.8)^0 = 10). For the reason that base of the exponential, (0.8), is lower than one, then (y) ought to lower because it takes on bigger values of (x). |
| 6. | D | 
start{align*} |
| 7. | A | At (x = -2), the curve has a minimal turning level so (f”(-2) > 0). At (x = 0), the curve attains a destructive y-intercept, so (f(0) < 0). At (x = 3), the curve has a most turning level, so (f'(3) = 0). Ordering from smallest to largest, now we have (f(0) < f'(3) < f”(-2).) |
| 8. | C | The graph of (y = f(x)) has a single root at (x = -1) and a triple root at (x = 3), so the type of the polynomial is (y = a(x + 1)(x – 2)^3). The behaviour of the curve on the extremities implies the main coefficient have to be destructive, which makes (C) the answer. |
| 9. | B | The spinoff of (h(x) = f(g(x))) is (h'(x) = f'(g(x)) g'(x)) by the chain rule. We’re on condition that the tangent to (y = h(x)) at (x = okay) has the equation (y = mx + c), so the spinoff at (x = okay) have to be the gradient. That’s, (h'(okay) = m). The gradient of the brand new tangent at (x = -k) isbegin{align*} h'(-k) &= f'(g(-k)) g'(-k) &= f'(g(okay)) g'(-k) & g(x) textual content{ is even means } g(-x) = g(x) &= f'(g(okay)) [-g'(k)] & textual content{Spinoff of a fair perform is odd} &= -h'(okay) &= -m finish{align*}For the reason that tangent at (x = okay) should intersect (y = h(x)), then (h(okay) = mk + c).start{align*} h(-k) &= f(g(-k)) &= f(g(okay)) &= mk + c finish{align*}We are able to use point-gradient method right here,start{align*} y – (mk + c) &= -m(x + okay) y – mk – c &= -mx – mk y &= -mx + c finish{align*} |
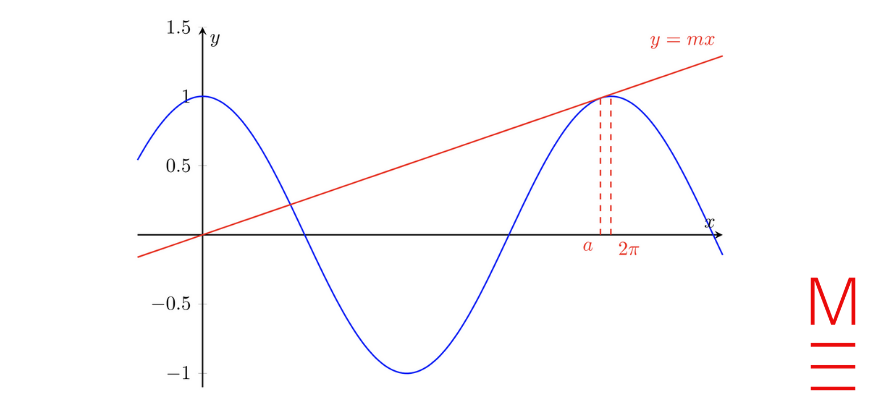
| 10. | B | The general image is
Zooming into the place the tangent touches the (y = cos x) curve,
By contemplating the gradients of the above traces, we then have the ordering (frac{1}{2pi} < m < frac{1}{a}) |
Lengthy Response Questions
Query 11
| start{align*} x + frac{x – 1}{2} &= 9 2x + x – 1 &= 18 3x &= 19 x &= frac{19}{3} finish{align*} |
Query 12a
| start{align*} cos 30^circ &= frac{XY}{16} XY &= 16 cos 30^circ &= 16 (frac{sqrt{3}}{2}) &approx 13.86 ; textual content{cm} textual content{(2 d.p.)} finish{align*} |
Query 12b
| start{align*} textual content{Space of semicircle} &= frac{1}{2} occasions pi(8)^2 &= 32pi ; textual content{cm}^2 finish{align*} |
Utilizing the Pythagorean theorem, we are able to full the triangle as
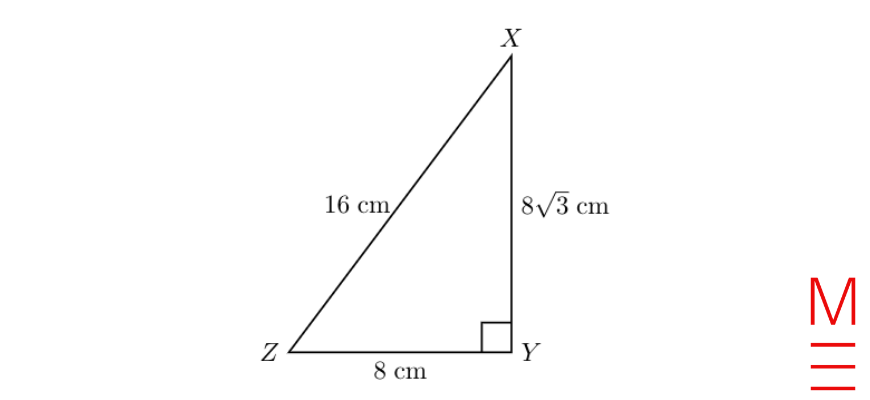
| start{align*} textual content{Space of triangle} &= frac{1}{2}(8)(8 sqrt{3}) &= 32 sqrt{3} ; textual content{cm}^2 finish{align*}start{align*} textual content{Whole space of shaded area} &= textual content{Space of semicircle} – textual content{Space of triangle} &= 32 pi – 32 sqrt{3} &approx 45.1 ; textual content{cm}^2 textual content{(1 d.p.)} finish{align*} |
Query 13
| start{align*} y &= x tan(x) frac{dy}{dx} &= 1 occasions tan(x) + x occasions sec^2(x) &= tan(x) + x sec^2(x) finish{align*}At (x = frac{pi}{3}),start{align*} frac{dy}{dx} &= tan(frac{pi}{3}) + frac{pi}{3} sec^2(frac{pi}{3}) &= sqrt{3} + frac{pi}{3} occasions frac{1}{frac{1}{4}} &= sqrt{3} + frac{4pi}{3} finish{align*}(∴ textual content{gradient of tangent at } x = frac{pi}{3} textual content{ is } sqrt{3} + frac{4pi}{3}) |
Query 14
| start{align*} S_n &= frac{n}{2} huge(2 a + (n-1) dbig) 2021 &= frac{43}{2} huge(2 occasions 5 + (43-1)dbig) 94 &= 10 + 42d d &= frac{94 – 10}{42} &= 2 finish{align*} |
Query 15
| start{align*} int_{-2}^0 sqrt{2x + 4} ; dx &= int_{-2}^0 (2x + 4)^{frac{1}{2}} ; dx &= frac{2}{3} occasions frac{1}{2} left[(2x + 4)^{frac{3}{2}}right]_{-2}^0 &= frac{1}{3} [4^{frac{3}{2}} – 0] &= frac{8}{3} finish{align*} |
Query 16
|
(f(x) textual content{ is rising when } f'(x) > 0.) start{align*} (2x(1-3x) geq 0) |
Query 17 a
(i)
| start{align*} y &= 29.2 – 0.011(540) &= 23.26^circ textual content{C} &approx 23.3^circ textual content{C} textual content{(1 d.p.)} finish{align*} |
(ii)
As the peak of the city above sea degree will increase, the typical most every day temperature decreases. Particularly, for each metre above sea degree, there’s roughly a (0.011^circ textual content{C}) lower within the common most every day temperature. Because the correlation coefficient is 0.494, there’s a average destructive correlation of this knowledge.
Query 17b
Latitude can be a greater predictor of the city’s common every day most temperature as there’s a stronger destructive correlation for this knowledge of (r = -0.897) versus solely a average destructive correlation coefficient of (-0.494) given by the comparability of the peak above sea degree and common every day most temperature.
Query 18
Utilizing sine rule in (triangle ABC), let (angle ABC = alpha).
| start{align*} frac{sin(alpha)}{25} &= frac{sinleft(28^circright)}{16} sin(alpha) &= frac{25}{16} sin(28^circ) ∴alpha &= sin^{-1}left(frac{25}{16} sin(28^circ)proper) finish{align*}The 2 options are,start{align*} ∴ alpha &= sin^{-1}left(frac{25}{16} sin(28^circ)proper) textual content{, } 180^circ – sin^{-1}left(frac{25}{16} sin(28^circ)proper). finish{align*}Since (alpha) is obtuse,start{align*} ∴ alpha &= 180^circ – sin^{-1}left(frac{25}{16} sin(28^circ)proper) &approx 133^circ ; textual content{(nearest diploma)} finish{align*} |
Query 19
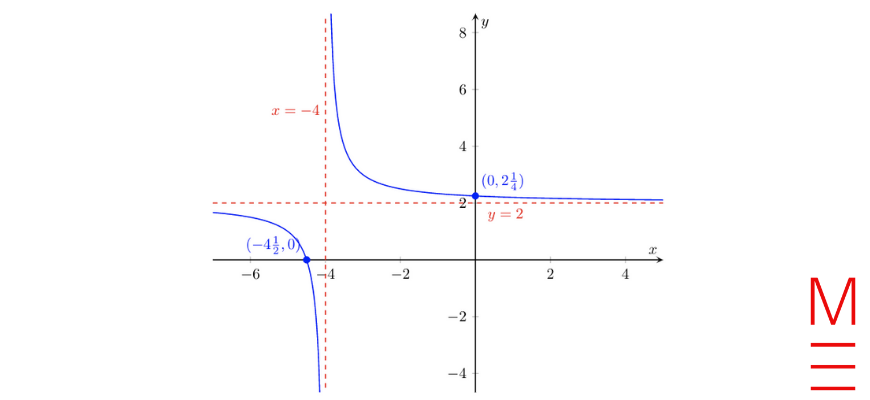
The graph of (y = 2 + frac{1}{x+4}) is:
Query 20
| start{align*} 2 sin(4x) &= 1 sin(4x) &= frac{1}{2} finish{align*}To unravel for (0 leq x leq frac{pi}{4}), we have to clear up the above trigonometric equation for (0 leq 4x leq pi).start{align*} ∴ 4x &= frac{pi}{6}, frac{5pi}{6}. ∴ x &= frac{pi}{24}, frac{5pi}{24}. finish{align*} |
Query 21
The turning two turning factors are at (0,0) and (2, -32) and (x)-intercepts at 0 and three.
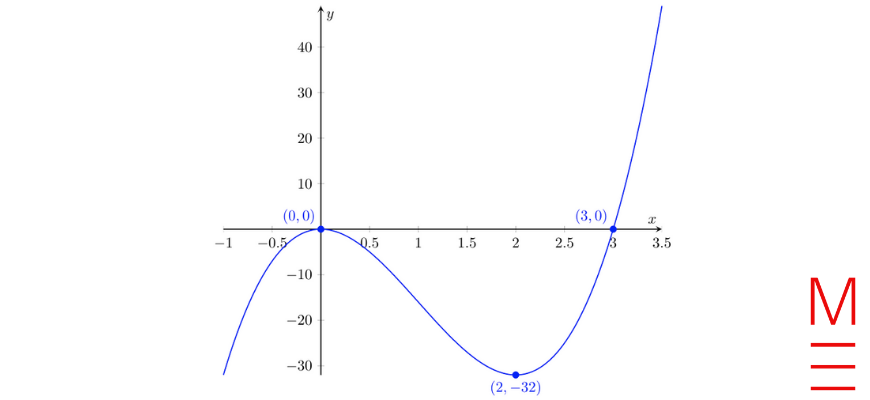
Query 22a
| start{align*} & 0.1915 – 0.0398 = 0.1517 finish{align*} |
Query 22b
| start{align*} & P(W > 3528) &= P(Z > frac{3528 – 3300}{570}) &= P(Z > 0.4) &= 1 – P(Z leq 0) – P(0 < Z < 0.4) &= 1 – frac{1}{2} – 0.1554 &= 0.3446. finish{align*}start{align*} ∴ textual content{Anticipated variety of infants out of } 1000 &= 1000 occasions 0.3446 &= 345 textual content{(nearest entire child)} finish{align*} |
Query 23
First clear up for (b) by substituting (P = 1250) and (t = 20),
| start{align*} 1250 &= 5000b^{-frac{20}{10}} frac{1}{4} &= b^{-2} b^2 &= 4 ∴b &= 2 textual content{ since } b textual content{ is constructive}. finish{align*}Differentiate (P(t)) to be able to discover (t) when (frac{dP}{dt} = -30).start{align*} frac{dP}{dt} &= frac{5000 occasions ln{2} occasions 2^{frac{-t}{10}}}{-10} &= -500 occasions ln{2} occasions 2^{frac{-t}{10}} finish{align*}Let (frac{dP}{dt} = -30) and clear up for (t),start{align*} -30 &= -500 occasions ln{2} occasions 2^{frac{-t}{10}} 2^{frac{-t}{10}} &= frac{30}{500 occasions ln{2}} frac{-t}{10} &= log_2 left(frac{30}{500 occasions ln{2}}proper) ∴ t &= -10 log_2 left(frac{30}{500 occasions ln{2}}proper) ∴ t &approx 35.3 textual content{ years (1 d.p.)}. finish{align*} |
Query 24
| start{align*} textual content{Shaded space} &= frac{1}{2}(2)(3) + int_{2}^4 frac{3}{x – 1} ; dx &= 3 + 3 left[ln{(x-1)}right]_{2}^{4} &= 3 + 3left[ln{(3)} – ln{(1)}right] &= 3 + 3ln{(3)} &= 3 + ln{(27)} ; textual content{items}^2 finish{align*} |
Query 25
Utilizing the desk of future worth curiosity elements for the primary 8 years,
| start{align*} A &= 8.2132 occasions 1000 &= 8213.2 finish{align*}Leaving the cash in her financial savings account for an additional 2 years at 1.25%,start{align*} A &= 8213.2 occasions (1.0125)^2 &approx $ 8419.81 textual content{(2 d.p.)}. finish{align*} |
Query 26a
To seek out the utmost top, first differentiate (y(t)) with respect to (t).
| start{align*} y(t) &= -5t^2 + 70t + 100 y'(t) &= -10t + 70 y”(t) &= -10 finish{align*} Stationary level is attained when (y'(t) = 0), so substituting in, start{align*} y'(t) &= 0 -10t + 70 &= 0 ∴ t &= 7 finish{align*} Confirm that (t = 7) is a most as a result of (y”(7) = -10 < 0).Therefore, the utmost top reached by the particle is,start{align*} y(7) &= -5(7)^2 + 70(7) + 100 y(7) &= 345 textual content{ m} finish{align*} |
Query 26b
So as to discover the speed, i.e. (y'(t)) of the particle earlier than hitting the bottom. First discover (t) when the particle has a top of zero, i.e. (y(t) = 0).
| start{align*} y(t) &= 0 -5t^2 + 70t + 100 &= 0 textual content{(use quadratic method)} ∴ t &= 7 pm sqrt{69} finish{align*}Since (7 – sqrt{69} < 0), then the particle hits the bottom when (t = 7 + sqrt{69}). Then, we are going to solely discover (y'(7 + sqrt{69})),start{align*} y'(7 + sqrt{69}) &= -10(7 + sqrt{69}) + 70 &= -70 – 10sqrt{69} + 70 &= – 10sqrt{69} finish{align*}(∴textual content{ velocity } = -10 sqrt{69} ; textual content{m}^2) |
Query 27a
The turning level under is at (6,400) the place the x-intercepts are at 0 and 12.
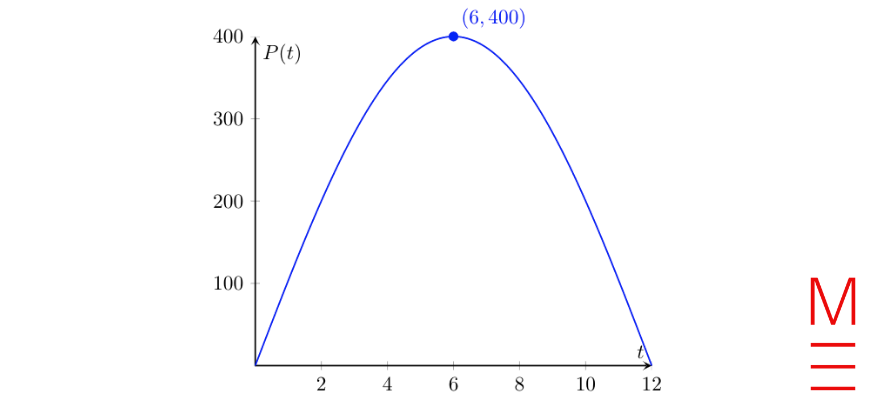
Query 27b
| start{align*} E &= int_{a}^{b} P(t) ; dt &= int_{a}^{b} 400 sinleft( frac{pi}{12} tright) ; dt &= frac{400 occasions 12}{pi} left[ -cosleft( frac{pi}{12}tright)right]_{a}^{b}; dt &= frac{4800}{pi} left[ cosleft( frac{api}{12}right) – cosleft( frac{bpi}{12}right)right] finish{align*} |
Query 27c
Use half (b) and let (a = 3) and (E=300), then clear up for (b).
| start{align*} 300 &= frac{4800}{pi} left[ cosleft( frac{3pi}{12}right) – cosleft( frac{bpi}{12}right)right] frac{300 pi}{4800} &= frac{1}{sqrt{2}}- cosleft( frac{bpi}{12}proper) cosleft( frac{bpi}{12}proper) &= frac{1}{sqrt{2}} – frac{pi}{16} b &= frac{12}{pi} cos^{-1} left(frac{1}{sqrt{2}} – frac{pi}{16}proper) b &approx 3.95238 finish{align*}Therefore, ready time isbegin{align*} b – a &= 3.95238 – 3 &= 0.95238 ; textual content{hours} &approx 57.142 ; textual content{minutes} finish{align*}Subsequently, the least period of time that Kenzo should wait to the closest minute is 58 minutes. |
Query 27d
From the graph drawn partly (a), we are able to observe that the utmost energy output is attained 6 hours after dawn. Thus it could not require as lengthy for Kenzo to cost his cellphone ranging from 6 hours after dawn as in comparison with ranging from 3 hours after dawn.
Query 28a
First discover the x-intercept of the perform (f(x) = 8 – 2^x).
| start{align*} 0 &= 8 – 2^x 2^x &= 8 ∴ x &= 3 finish{align*}Subsequent, combine (f(x)) from 0 to three to be able to discover the realm below the curve.start{align*} textual content{Space} &= int_{0}^{3} 8 – 2^x ; dx &= left[ 8x – frac{2^x}{ln{(2)}}right]_{0}^{3} &= left(8(3) – frac{2^{(3)}}{ln{(2)}}proper) – left( 8(0) – frac{2^{(0)}}{ln{(2)}}proper) &= 24 – frac{8}{ln(2)} + frac{1}{ln(2)} &= 24 – frac{7}{ln{(2)}} finish{align*} |
Query 28b
The graph under has an x-intercept at 2 and asymptote of (y=-8).

Query 28c
| start{align*} int_{2}^{5} g(x) ; dx &= int_{-3}^{0} -f(-x) ; dx &= int_{0}^{3} -f(x) ; dx &= – int_{0}^{3} f(x) ; dx &= – left[ 24 – frac{7}{ln{(2)}}right] &= frac{7}{ln{(2)}} – 24 finish{align*} |
Query 29a
Let (A_n) be the quantity within the account on Megan’s n-th birthday.
| start{align*} A_1 &= 5000(1.03) + 1000 A_2 &= A_1(1.03) + 1000 = 5000(1.03)^2 + 1000(1.03) + 1000 A_3 &= A_2(1.03) + 1000 = 5000(1.03)^3 + 1000(1.03)^2 + 1000(1.03) + 1000 = $8554.54 finish{align*} |
Query 29b
We’re on condition that (A_{17} = 30 025.83).
Let (B_n) be the quantity within the account after the n-th annual withdrawal.
| start{align*} B_1 &= A_{17}(1.03) – 2000 B_2 &= A_{17}(1.03)^2 – 2000(1.03) – 2000 &vdots B_n &= A_{17}(1.03)^n – 2000[(1.03)^{n-1} + (1.03)^{n-2} + dots + 1.03 + 1] &= A_{17}(1.03)^n – frac{2000(1 – 1.03^n)}{1 – 1.03} finish{align*}Resolve for (B_n = 0),start{align*} A_{17}(1.03)^n – frac{2000(1 – 1.03^n)}{1 – 1.03} &= 0 A_{17}(1.03)^n – frac{2000}{-0.03} + frac{2000(1.03)^n}{-0.03} &= 0 left(A_{17} – frac{2000}{0.03}proper)(1.03)^n &= – frac{2000}{0.03} (1.03)^n &= – frac{2000}{0.03} occasions frac{1}{A_{17} – frac{2000}{0.03}} n &= frac{1}{ln(1.03)} ln left(-frac{2000}{0.03} occasions frac{1}{A_{17} – frac{2000}{0.03}}proper) &approx 20.249 finish{align*}Therefore, Megan could make a most of 20 withdrawals of ($2,000) earlier than her account stability is inadequate. |
Query 30
Resolve for (x) when (F(x) = 0.99)
| start{align*} 0.99 &= 1- e^{-0.01x} e^{-0.01x} &= 0.01 -0.01x &= frac{ln{(0.01)}}{-0.01} ∴ x &= 460.52 textual content{ hours (2 d.p.)} finish{align*} |
Query 31
| start{align*} y &= x^2 – 1 frac{dy}{dx} &= 2x finish{align*}Equate the gradient of the tangent when (x = a) with the gradient of the road that passes via ((3, -8)) and ((a, a^2-1)),start{align*} frac{-8 – (a^2 – 1)}{3 – a} &= 2(a) -8 – a^2 + 1 &= 6a – 2a^2 a^2 – 6a -7 &= 0 (a – 7)(a + 1) &= 0 ∴ a &= 7, -1 finish{align*}Subsequently, the 2 factors on (y = x^2-1) the place their tangents go via ((3,-8)) are (A(7,48)) and (B(-1, 0)). Utilizing the point-gradient method, the equation of the tangent at (A(7,48)) isbegin{align*} y – 48 &= 14(x – 7) y &= 14x – 98 + 48 ∴ y &= 14x – 50 finish{align*}Equally for (B(-1,0)),start{align*} y – 0 &= -2(x + 1) ∴ y &= -2x – 2 finish{align*} |
Query 32
Let (X) be a feminine’s top within the inhabitants and (Y) be a male’s top.
| start{align*} P(X< 175) &= 97.5% P(X < 160.6) &= 16% P(Y < h) &= 84% finish{align*}Our goal is the discover (h). Utilizing the empirical rule with the knowledge given above.start{align*} mu + 2sigma &= 175 & textual content{(1)} mu – sigma &= 160.6 & textual content{(2)} finish{align*}Subtracting equation (1) by (2) givesbegin{align*} 3 sigma &= 14.4 ∴ sigma &= 4.8 finish{align*}Substitute (sigma = 4.8) into (1),start{align*} mu + 2(4.8) &= 175 mu = 165.4 finish{align*}Therefore, for the grownup male inhabitants, the imply is (1.05 mu = 173.67) and the usual deviation is (1.1 sigma = 5.28). Since being (84%) taller than the male inhabitants is 1 normal deviation above the imply, then we are able to calculate (h) asbegin{align*} h &= 1.05 mu + 1.1 sigma &= 173.67 + 5.28 &= 178.95 ; textual content{cm} finish{align*} |
Query 33a
Utilizing the truth that the entire likelihood is all the time (1),
| start{align*} int_{-infty}^{infty} f(x) ; dx &= 1 int_{0}^{6} frac{Ax}{x^2 + 4} ; dx &= 1 A left[frac{1}{2} ln{(x^2 + 4)}right]_{0}^{6} &= 1 frac{A}{2}left[ln(40) – ln(4)right] &= 1 finish{align*}start{align*} ∴ A &= frac{2}{ln{(40)} – ln{(4)}} &= frac{2}{ln(frac{40}{4})} &= frac{2}{ln(10)} finish{align*} |
Query 33b
Discover when the primary spinoff is the same as zero to search out the utmost turning level.
The primary spinoff is
| (frac{dy}{dx} = frac{2}{ln{(10)}} left[frac{4 – x^2}{(x^2+4)^2} right]) Resolve for (frac{dy}{dx} = 0),start{align*} frac{2}{ln{(10)}} left[frac{4 – x^2}{(x^2+4)^2} right] &= 0 4 – x^2 &= 0 x &= pm 2 ∴x &= 2 textual content{ since } x in [0, 6] finish{align*} |
Query 33c
| start{align*} P(X < 2) &= int_{0}^{2} frac{Ax}{x^2 + 4} ; dx &= frac{2}{ln{(10)}}left[frac{1}{2} ln{(x^2+4)} right]_{0}^{2} &= frac{1}{ln{(10)}} left[ln{left(2^2 + 4right)} – ln{left(0^2 + 4right)} right] &= frac{1}{ln{(10)}} left[ln{(8)} – ln{(4)} right] &= frac{ln{left(frac{8}{4} proper)}}{ln{(10)}} &= frac{ln{(2)}}{ln{(10)}} ∴ P(X <2) &= log_{10}{(2)} textual content{(utilizing change of base rule)} finish{align*} |
Query 33d
We are able to write the next information through the use of the empirical rule and outcomes from earlier components:
| start{align*} &P(IQ > 130) = 2.5% = 0.025 &P(X < 2) = log_{10}{(2)} &P(X < 2 | IQ > 130) = 80% = 0.8 finish{align*}The goal is to search out the likelihood that an individual has an IQ larger than 130, on condition that they’ll full the puzzle in lower than two hours. That’s, we need to discover (P(IQ > 130 | X < 2)),start{align*} P(IQ > 130 | X < 2) &= frac{P(IQ < 130 textual content{ and } X < 2)}{P(X < 2)} &= frac IQ > 130) occasions P(IQ > 130){P(X < 2)} &= frac{0.8 occasions 0.025}{log_{10}(2)} &approx 0.066 textual content{(3 d.p.)} finish{align*} |
Query 34
| start{align*} Eleft(Xright) &= r occasions r^n + r^2 occasions r^{n – 1} + … + r^{okay} occasions r^{n – okay + 1} + .. + r^n occasions r Eleft(Xright) &= r^{n + 1} + r^{n + 1} + … + r^{n + 1} textual content{ (the summation is $r^{n + 1}$ summed $n$ occasions)} Eleft(Xright) &= n(r^{n + 1}) … (1) finish{align*}Additionally word that the summation of the possibilities equate to at least one.start{align*} sum_{x}P(X = x) &= 1 r^n + r^{n – 1} + … + r &= 1 frac{r occasions (1 – r^n)}{1 – r} &= 1 ∴ r^{n+1} &= 2r – 1 … (2) finish{align*}By substituting equation (2) into equation (1),start{align*} ∴ Eleft(Xright) &= n(2r – 1) finish{align*} |
,