The 2019 HSC Mathematics Advanced (2 Unit) exam paper solutions are here!
2019 HSC Maths Advanced Exam Paper Solutions
Have you seen the 2019 HSC Mathematics Advanced (2 Unit) exam paper yet?
In this post, we will work our way through the 2019 HSC Maths Advanced (2 Unit) paper and give you the solutions, written by our Head of Mathematics Oak Ukrit and his team.
Read on to see how to answer all of the 2019 questions.
Section 1. Multiple Choice
| Question Number | Answer | Solution |
| 1. | B | \(\) \begin{align*} \pi^{10} &= 93648.0478\\ &= 9.3648\times10^4\\ &= 9.4 \times 10^4 \textrm{ (2 s.f.)}\\ \end{align*} |
| 2. | D | \begin{align*} -3x &\leq 8\\ x &\geq -\frac{8}{3} \end{align*} |
| 3. | C | \begin{align*} \frac{a^2a^{-3}}{\sqrt{a}} &= \frac{a^2a^{-3}}{a^\frac{1}{2}}\\ &= a^{2-3-1/2}\\ &= a^{-3/2}\\ ∴ a^p &= a^{-3/2} \\ ∴ p &= -\frac{3}{2} \end{align*} |
| 4. | C | As the focus is to the right of the vertex, it must be a positive sideways parabola. As the vertex is at \((2,1)\), the equation must be of the form \((y-1)^2 = 4a(x-2)\). Thus the only possible solution is \(C\). |
| 5. | A | \begin{align*} \frac{\log_29}{\log_23} &= \frac{\log_23^2}{\log_23}\\ &= \frac{2\log_23}{\log_23}\\ &= 2 \end{align*} |
| 6. | C | \begin{align*} \textrm{P(winning)} &= 1 – \textrm{P(losing)} \\ &= 1 – \textrm{P(odd number)}\cdot\textrm{P(heads)} \\ &= 1 – \frac{1}{2}\cdot\frac{1}{2} \\ &= \frac{3}{4} \end{align*} |
| 7. | D | It can be seen that the range of the curve is \(3\) units, which means the curve is stretched \(1.5\) times vertically (\(a = 1.5\)). The curve also has a period of \(\pi\), which means that the curve has been squished 2 times horizontally (\(b = 2\)). |
| 8. | C | A maximum will occur at time \(t\), when the derivative at time \(t\) is \(0\), and the derivative is positive just before, and negative just after. As acceleration is the derivative of velocity, by the graph this is only satisfied at \(t = 3\). |
| 9. | A | \begin{align*} \int\tan^2x\,dx &= \int(\sec^2x-1)dx\\ &= \tan x – x + C \end{align*} |
| 10. | C | Although this question is easier to do by showing why the other 3 choices are false, this is why C is true.
Consider the velocity function. We know that it will equal \(0\) at \(t = 11\) and \(t=13\). We are trying to show that the velocity function must have a stationary point in between \(t = 11\) and \(t = 13\). Assume that it doesn’t have a stationary point. Therefore the velocity function must either be always increasing, or always decreasing from \(t = 11\) to \(t=13\) as it can never switch. However if this is the case, the velocity function can never come back to \(0\). Therefore this is impossible and it must have a stationary point. |
Section 2. Long response
Question 11a
\begin{align*}
\frac{x}{\sin(40°)} &= \frac{8}{\sin(110°)}\\
x &= \frac{8\sin(40°)}{\sin(110°)}\\
&= 5.5 \textrm{ units (1 d.p)}
\end{align*}
Question 11b
| \(u = x^2\) | \(v = \sin x\) |
| \(u’ = 2x\) | \(v’ = \cos x\) |
\begin{align*}
∴ \frac{d}{dx}(x^2\sin x) &= (2x)(\sin x) + (x^2)(\cos x) \\
&= 2x\sin x + x^2\cos x \\
\end{align*}
Question 11c
| \(u = 2x + 1\) | \(v = x + 5\) |
| \(u’ = 2\) | \(v’ = 1\) |
\begin{align*}
\frac{d}{dx}\frac{2x+1}{x+5} &= \frac{(2)(x+5)-(2x+1)(1)}{(x+5)^2}\\
&= \frac{2x+10-2x-1}{(x+5)^2}\\
&= \frac{9}{(x+5)^2}
\end{align*}
Question 11d
\begin{align*}
a &=2000, r=\frac{-1200}{2000} = -\frac{3}{5}\\
∴ S_\infty &= \frac{2000}{1-(-\frac{3}{5})}\\
&= 1250
\end{align*}
Question 11e
\begin{align*}
\int_0^1 \frac{1}{(3x+2)^2}dx &= \int_0^1 (3x+2)^{-2} dx\\
&= \frac{1}{3}\left[\frac{(3x+2)^{-1}}{-1}\right]_0^1\\
&= -\frac{1}{3}\left[5^{-1}-2^{-1}\right]\\
&= \frac{1}{10}
\end{align*}
Question 11f
\begin{align*}
\textrm{P(2 green or 2 purple)} &= \textrm{P(2 green) + P(2 purple)}\\
&= \frac{5}{12}\times\frac{4}{11} + \frac{7}{12}\times\frac{6}{11}\\
&= \frac{31}{66}
\end{align*}
Question 11g
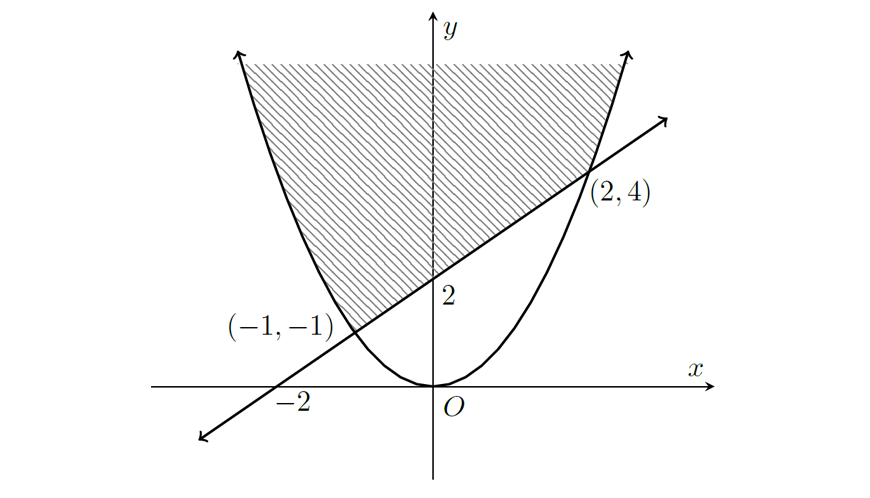
Question 12a
Part (i)
\begin{align*}
\textrm{For line AB:}\\
x-2y+4&=0\\
2y &= x+4\\
y &= \frac{1}{2}x + 2\\
∴ m_{AB} &= \frac{1}{2}\\
∴ m_{AC} &= -2\\
\textrm{AC passes through (8,6)}
∴ y-6 &= -2(x-8)\\
y-6 &= -2x+16\\
∴ AC: 2x+y-22 &= 0
\end{align*}
Part (ii)
\begin{align*}
\textrm{For line AC, let } y = 0:\\
∴ 2x-22 &=0\\
x &= 11\\
∴ C(11,0)\\
\textrm{For line AB, let } y = 0 :\\
∴ x + 4 &= 0\\
x &= -4\\
∴ B(-4,0)\\
∴ BC &= 15\\
\textrm{height } &= 6\\
∴ \textrm{Area}_\textrm{triangle} &= \frac{1}{2}(15)(6)\\
&= 45 \textrm{ units}^2
\end{align*}
Question 12b
\begin{align*}
T_4 = a + 3d &= 6\\
a &= 6-3d\\
S_{16} = \frac{16}{2}(2a + 15d) &= 120\\
8(2(6-3d)+15d) &=120\\
12-6d+15d &= 15\\
9d &= 3\\
d &= \frac{1}{3}
\end{align*}
Question 12c
Part (i)
\begin{align*}
200000e^{-0.14\times31} = 2607 \textrm{ leaves (rounded down to nearest whole number)}
\end{align*}
Part (ii)
\begin{align*}
L(t) &= 200000e^{-0.14t}\\
L'(t) &= 200000(-0.14)e^{-0.14t}\\
&= -28000e^{-0.14t}\\
L'(31) &= -28000e^{-0.14\times31}\\
&= -365.02 \textrm{ leaves per day (2 d.p)} \\
& ∴ \textrm{365 leaves fall per day}
\end{align*}
Part (iii)
\begin{align*}
200000e^{-0.14t} &= 100\\
e^{-0.14t} &= \frac{1}{2000}\\
-0.14t &= \ln \frac{1}{2000}\\
t &= \frac{1}{-0.14}\ln \frac{1}{2000}\\
&= 54.29 \textrm{ days (2 d.p)} \\
&∴ \textrm{55th day} \\
\end{align*}
Question 12d
\begin{align*}
A &= \int_0^3\frac{3x}{x^2+1}dx\\
&= \frac{3}{2} \int_0^3\frac{2x}{x^2+1}dx\\
&=\frac{3}{2} \left[\ln(x^2+1)\right]_0^3\\
&= \frac{3}{2}\ln10 \textrm{ units}^2
\end{align*}
Question 13a
\begin{align*}
2\sin x\cos x &= \sin x &&\\
2\sin x\cos x – \sin x &= 0 &&\\
\sin x(2\cos x-1) &= 0\\ &&\\
\sin x &= 0 & 2\cos x -1 &= 0\\
x &= 0, \pi, 2\pi & 2\cos x &= 1\\
&& \cos x &= \frac{1}{2}\\
&& x &= \frac{\pi}{3},\frac{5\pi}{3}\\
∴ x &= 0, \frac{\pi}{3},\pi,\frac{5\pi}{3},2\pi
\end{align*}
Question 13b
\begin{align*}
\textrm{Perimeter} = \textrm{line AB} + \textrm{arc AB}\\
OA = OB \textrm{ (radii of circle)}\\
∴\Delta OAB \textrm{ is isoceles}\\
∴ \angle OBA = \angle BAO &= \frac{180°-70°}{2}\\
&= 55°\\
\textrm{Applying sine rule to }&\Delta OAB:\\
\frac{\textrm{line AB}}{\sin(70°)} &= \frac{20}{\sin(55°)}\\
\textrm{line AB} &= \frac{20\sin(70°)}{\sin(55°)}\\
\textrm{Also:}\\
\textrm{arc AB} &= r\theta\\
&= 20\times\frac{70°\times\pi}{180°}\\
∴ \textrm{Perimeter} &= \frac{20\sin(70°)}{\sin(55°)} + 20\times\frac{70°\times\pi}{180°}\\
&= 47.4 \textrm{ cm (1 d.p)}
\end{align*}
Question 13c
Part (i)
\begin{align*}
\frac{d}{dx}(\ln x)^2 &= 2\ln x \times \frac{1}{x}\\
&= \frac{2\ln x}{x}
\end{align*}
Part (ii)
\begin{align*}
\frac{d}{dx}(ln x)^2 &= \frac{2\ln x}{x}\textrm{ (from (i))}\\
∴ \int \frac{2\ln x}{x}dx &= (\ln x)^2 + C\\
∴ \int \frac{\ln x}{x}dx &= \frac{1}{2}(\ln x)^2 + C
\end{align*}
Question 13d
\begin{align*}
V &= \pi\int_a^by^2dx\\
&= \pi\int_0^1(x-x^3)^2dx\\
&= \pi\int_0^1(x^2-2x^4+x^6)dx\\
&= \pi\left[\frac{x^3}{3}-\frac{2x^5}{5}+\frac{x^7}{7}\right]_0^1\\
&= \pi\left(\frac{1}{3}-\frac{2}{5}+\frac{1}{7}\right)\\
&=\frac{8\pi}{105} \textrm{ units}^3
\end{align*}
Question 13e
Part (i)
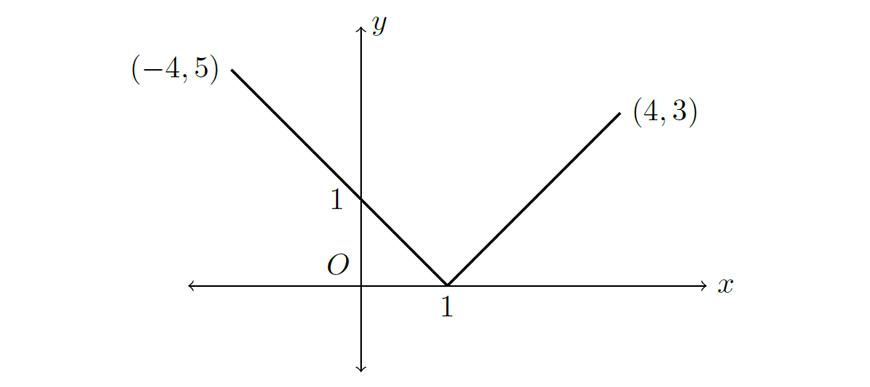
Part (ii)

From the sketch, there is only one solution for \(2x+4 = |x-1|\), which occurs on the negative branch.
\begin{align*}
∴2x+4 &= 1-x\\
3x &= -3\\
x &= -1
\end{align*}
Question 14a
\begin{align*}
\frac{dv}{dt} &= e^{2t}-4\\
v &= \frac{1}{2}e^{2t}-4t + C\\
\textrm{At }t &= 0, v=0:\\
∴ 0 &= \frac{1}{2} + C\\
C &= -\frac{1}{2}\\
∴ v&= \frac{1}{2}e^{2t}-4t-\frac{1}{2}
\end{align*}
Question 14b
Part (i)
\begin{align*}
f'(x) &= 3x^2+2x-1\\
\textrm{Let } f'(x) = 0:\\
∴ 3x^2+2x-1 &= 0\\
(x+1)(3x-1) &= 0\\
∴ x&=-1,\frac{1}{3}\\
f”(x) &= 6x + 2\\
f”(-1) &= -4 < 0\\
f”(\frac{1}{3}) &= 4 > 0\\
∴ \textrm{ minimum at } x&=\frac{1}{3}, \textrm{ maximum at } x=-1
\end{align*}
Part (ii)
\begin{align*}
f(x) &= x^3 + x^2 – x + C\\
f(0) &= 4\\
∴ C &= 4\\
∴ f(x) &= x^3 + x^2 – x + 4
\end{align*}
Part (iii)
\begin{align*}
\textrm{minimum at } \left(\frac{1}{3}, \frac{103}{27}\right), \textrm{ maximum at } (-1, 5)\\
\textrm{y-intercept at } (0,4)
\end{align*}
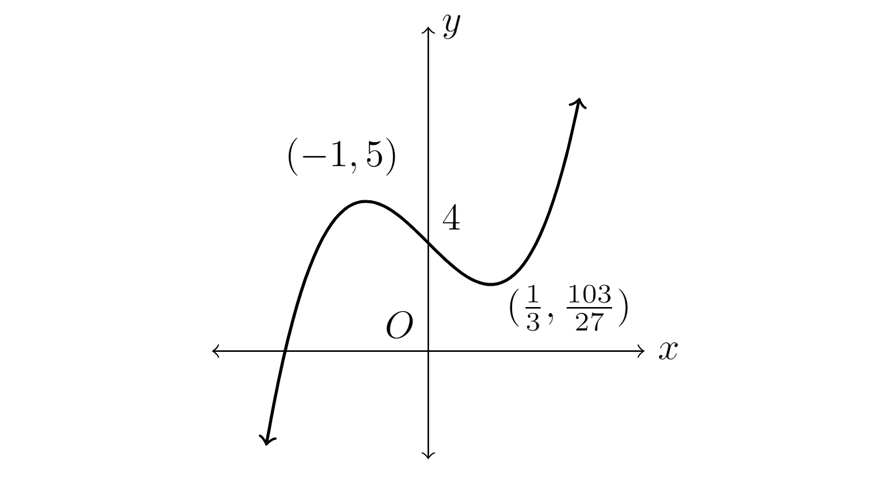
Part (iv)
\begin{align*}
f”(x) = 6x + 2 &< 0\\
6x < -2\\
x < -\frac{1}{3}
\end{align*}
Question 14c
The sum of the interior angles of hexagon is \((6-2)\times 180^{\circ} = 720^{\circ}\) and thus every interior angle of this regular hexagon is \(720^\circ \div 6 = 120^\circ\).
\begin{gather*}
\triangle AFE \text{ is isosceles} \quad (∵ AF = AE = 1) \\
\angle AFE = 120^\circ \quad (∵ \text{interior angle of hexagon}) \\
∴ \angle FAE + \angle AEF = 60^\circ \quad (∵ \text{angle sum of triangle}) \\
\angle FAE = \angle AEF \quad (∵ \text{base angles of isos. triangle are equal}) \\
∴ \angle AEF = 30^\circ \\
\angle AED = \angle DEF – \angle AEF = 120^\circ – 30^\circ = 90^\circ \\
∴ \triangle DEX \text{ is a right angled triangle} \\
\angle EDX = 180^\circ – 120^\circ = 60^\circ \quad (∵ \text{supplementary angles})
\end{gather*}
Therefore from applying trig ratios on \(\triangle DEX\) we have that:
\begin{gather*}
\tan 60^\circ = \dfrac{EX}{ED} = \dfrac{EX}{1} = EX \\
EX = \sqrt{3}
\end{gather*}
Question 14d
\begin{align*}
y &= x^3+ax^2+bx+4\\
\frac{dy}{dx} &= 3x^2+2ax+b\\
\textrm{At }x&=2, \frac{dy}{dx}=1\\
∴ 1 &= 12 + 4a + b\\
∴ 4a + b &= -11\quad\textrm{(1)}\\
\textrm{At} x &=2, y=-2\\
-2 &= 2^3 + 2^2(a) + 2b + 4\\
4a + 2b &= -14\quad\textrm{(2)}\\
\textrm{(2)} &- \textrm{(1)}\\
∴ b &= -3\\
∴ a &= -2
\end{align*}
Question 15a
\begin{align*}
e^{\ln x^2} &= x+6\\
x^2 &= x + 6\\
x^2 – x – 6 &= 0\\
(x-3)(x+2) &= 0\\
x &= 3, -2\\
\textrm{However, } x &> 0\\
∴ x &= 3
\end{align*}
Question 15b
\begin{align*}
CB &= \sqrt{q^2 + h^2}\\
AC &= \sqrt{p^2 + h^2}\\
AB^2 &= AC^2 + BC^2\\
∴ (p+q)^2 &= p^2 + h^2 + q^2 + h^2\\
p^2 + 2pq + q^2 &= p^2 + 2h^2 + q^2\\
2pq &= 2h^2\\
pq &= h^2\\
∴ h &= \sqrt{pq}\,\, (h>0 \textrm{ as h is a length})
\end{align*}
Question15c
Part (i)
\begin{align*}
\angle AQP = \angle PRB = 90° \textrm{ (given)}\\
RP\,&//\,AQ \textrm{ (given)}\\
∴ \angle QAB &= \angle RPB \textrm{ (corresponding angles on parallel lines)}\\
∴ \Delta PRB&\,|||\,\Delta AQP \textrm{ (equiangular)}\\
∴ \frac{x}{1} &= \frac{8}{BR} \textrm{ (corresponding sides of similar triangles in ratio)}\\
∴ BR &= \frac{8}{x}\\
\textrm{Extend BR and AQ to meet at S}\\
∴ BS &= \frac{8}{x} + 1\\
AS &= x+8\\
∴ D^2 &= \left(\frac{8}{x} + 1\right)^2 + (x+8)^2 \textrm{ as required}
\end{align*}
Part (ii)
\begin{align*}
D^2 &= (x+8)^2 + \left(\frac{8}{x} + 1\right)^2\\
\frac{d(D^2)}{dx} &= 2(x+8) + 2\left(\frac{8}{x}+1\right)\left(\frac{-8}{x^2}\right)\\
&= 2x+16 \ – \frac{16}{x^2}\left(\frac{8}{x}+1\right)\\
&= 2x+16 \ – \frac{128}{x^3}-\frac{16}{x^2}\\
&= 2x+16 \ – 128x^{-3}-16x^{-2}\\
\textrm{Sub } x=2:\\
\frac{d(D^2)}{dx} &= 2(2) + 16 \ – 128(2)^{-3}-16(2)^{-2}\\
&= 0\\
∴ &\textrm{ stationary point at } x=2\\
\frac{d^2(D^2)}{dx^2} &= 2 + 384x^{-4} + 32x^{-3}\\
\textrm{Sub } x=2:\\
\frac{d^2(D^2)}{dx^2} &= 2 + 384(2)^{-4} + 32(2)^{-3}\\
&= 30 > 0\\
∴ D^2 &\textrm{ is minimum at } x = 2
\end{align*}
Question15d
Part (i)
\begin{align*}
\textrm{P(at least 1 red hair for 2 people)} &= 1- \textrm{P(no red hair for 2 people)}\\
&= 1 – (0.98\times0.98)\\
&= 0.0396
\end{align*}
Part (ii)
\begin{align*}
\textrm{P(at least 1 red hair for n people)} &= 1- \textrm{P(no red hair for n people)}\\
&= 1- 0.98^n\\
∴ 1-0.98^n &> 0.4\\
0.6 &> 0.98^n\\
\ln{0.6} &> n\ln{0.98}\\
n &> \frac{\ln 0.6}{\ln 0.98}\\
&> 25.285…\\
& ∴ \textrm{at least 26 people}
\end{align*}
Question 16a
Part (i)
\begin{align*}
A_0 &= 1000000\\
A_1 &= 1000000\times(1+\frac{0.06}{4})^4-80000 = 1000000\times1.015^4-80000\\
A_2 &= (1000000\times1.015^4-80000)\times1.015^4 -80000\\
&= 1000000\times1.015^8 -80000(1+1.015^4)
\end{align*}
Part (ii)
\begin{align*}
A_n &= 1000000\times1.015^{4n} – 80000(1+1.015^4+1.015^8+…+1.015^{4(n-1)})\\
&= 1000000\times1.015^{4n} – 80000\left(\frac{1.015^{4n}-1}{1.015^4-1}\right)\\
&= 1000000\times1.015^{4n} – \frac{80000}{1.015^4-1}(1.015^{4n})+\frac{80000}{1.015^4-1}\\
&= 1.015^{4n}\left(1000000-\frac{80000}{1.015^4-1}\right)+\frac{80000}{1.015^4-1}\\
\textrm{Let }A_n = 0:\\
∴ 0 &= 1.015^{4n}\left(1000000-\frac{80000}{1.015^4-1}\right)+\frac{80000}{1.015^4-1}\\
-\frac{80000}{1.015^4-1} &= 1.015^{4n}\left(1000000-\frac{80000}{1.015^4-1}\right)\\
\frac{-\frac{80000}{1.015^4-1}}{1000000-\frac{80000}{1.015^4-1}} &= 1.015^{4n}\\
4.29266 &= 1.015^{4n}\\
\log_{1.015}4.29266 &= 4n\\
n &= \frac{1}{4}\times\frac{\ln4.29266}{\ln1.015}\\
&= 24.46\\
∴ 24 \textrm{ years}
\end{align*}
Question 16b
\begin{align*}
\textrm{Particle is at rest when }v&=0:\\
∴ 0 &= e^{\cos t}-1\\
1 &= e^{\cos t}\\
0 &= \cos t\\
∴ t &= \frac{\pi}{2} \textrm{ (take first solution)}\\
\end{align*}
\begin{align*}
∴ \textrm{ One application of Simpson’s rule takes points at } t=0,\frac{\pi}{4},\frac{\pi}{2}\\
\textrm{and } v= e-1, e^{\frac{1}{\sqrt{2}}}-1, 0 \textrm{ respectively}\\
\end{align*}
| \(t\) | \(0\) | \(\frac{\pi}{4}\) | \(\frac{\pi}{2}\) |
| \(v\) | \(e-1\) | \(e^{\frac{1}{\sqrt{2}}}-1\) | \(0\) |
\begin{align*}
∴ x &= \frac{\frac{\pi}{4}}{3}(e-1+0+4(e^{\frac{1}{\sqrt{2}}}-1))\\
&= 1.53 \textrm{ units to the right of the origin} \textrm{ (2 d.p)}
\end{align*}
Question 16c
Part (i)
\begin{align*}
y&=x^r\\
\frac{dy}{dx} = rx^{r-1}\\
\textrm{At }x=1:\\
\frac{dy}{dx} = r\\
∴ \textrm{equation of tangent:}\\
y-1&=r(x-1)\\
\textrm{At }y=0:\\
-1&=r(x-1)\\
\frac{-1}{r} &= x-1\\
x &= 1-\frac{1}{r}\\
&= \frac{r-1}{r} \textrm{ as required}
\end{align*}
Part (ii)
\begin{align*}
R &= \int_0^1x^r\,dx – A_\Delta\\
&= \left[ \frac{x^{r+1}}{r+1}\right]_0^1 – \frac{1}{2}(1-\frac{r-1}{r})(1)\\
&= \frac{1}{r+1} -\frac{1}{2r}\\
&= \frac{2r-(r+1)}{2r(r+1)}\\
&= \frac{r-1}{2r(r+1)} \textrm{ as required}
\end{align*}
Part (iii)
\begin{align*}
R &= \frac{r-1}{2r(r+1)}\\
&= \frac{r-1}{2r^2+2r}\\
\frac{dR}{dr} &= \frac{2r^2+2r – (r-1)(4r+2)}{(2r^2+2r)^2}\\
&= \frac{2r^2+2r – 4r^2 + 4r – 2r + 2}{(2r^2+2r)^2}\\
&= \frac{-2r^2+4r+2}{(2r^2+2r)^2}\\
\textrm{Let }\frac{dR}{dr} &= 0\\
∴ -2r^2+4r+2 &= 0\\
r^2-2r-1 &= 0\\
r &= \frac{2 \pm \sqrt{2^2-4(1)(-1)}}{2}\\
&= \frac{2 \pm \sqrt{8}}{2}\\
&= 1 \pm \sqrt{2}\\
∴ \textrm{ stationary points at } r = 1\pm \sqrt{2}
\end{align*}
| \(r\) | \(-0.5\) | \(1-\sqrt{2}\) | \(-0.1\) | \(0.1\) | \(1+\sqrt{2}\) | \(3\) |
| \(\frac{dR}{dr}\) | \(-2\) | \(0\) | \(48.77\) | \(49.17\) | \(0 \) | \( -0.0069\) |
| \(–\) | \(+\) | \(+\) | \(–\) |
(Note: the points chosen on either side of the stationary points must be continuous!)
\begin{align*}
∴ R \textrm{ is a maximum at } r = 1 + \sqrt{2}
\end{align*}
